Δυικός Χώρος
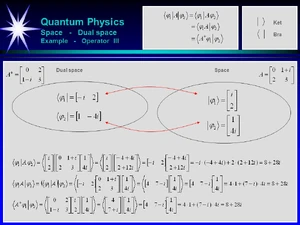
Δυικός Χώρος
Διανυσματική Βάση
Δυική Βάση
Γεωμετρία
Γραμμική Άλγεβρα
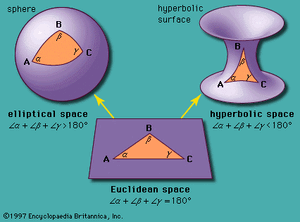
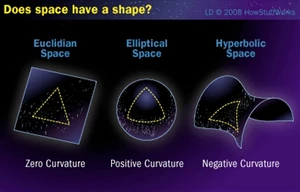
Γεωμετρικός Χώρος
Ευκλείδειος Χώρος
Χώρος Minkowski
Χώρος Riemann
Χώρος Lobachevsky
Μαθηματικός Χώρος
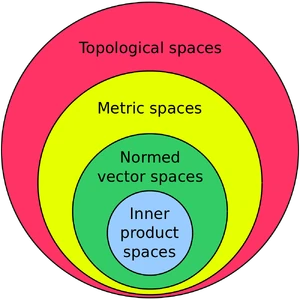
Τοπολογικός Χώρος
Διανυσματικός Χώρος
Μετρικός Χώρος
Χώρος Hilbert

Μοναδικότητα Δυικότητα Τριαδικότητα Τετραδικότητα
Μοναδικότητες Δυικότητες Τριαδικότητες Τετραδικότητες
Συμπληρωματικότητα
Φιλοσοφικός Δυισμός Φιλοσοφικές Δυικότητες
Μαθηματικός Δυισμός Μαθηματικές Δυικότητες
Πληροφορικός Δυισμός Πληροφορικές Δυικότητες
Φυσικός Δυισμός Φυσικές Δυικότητες
Χημικός Δυισμός Χημικές Δυικότητες
Γεωλογικός Δυισμός Γεωλογικές Δυικότητες
Βιολογικός Δυισμός Βιολογικές Δυικότητες
Οικονομικός Δυισμός Οικονομικές Δυικότητες
Κοινωνικός Δυισμός Κοινωνικές Δυικότητες
Αστρονομικός Δυισμός Αστρονομικές Δυικότητες
Δυικός Χώρος Δυικό Πολύπτυχο Δυική Βάση Δυική Αναπαράσταση
- Ένας Μαθηματικός Χώρος.
Ετυμολογία[]
Η ονομασία "Δυικός" σχετίζεται ετυμολογικά με την λέξη "δύο".
Εισαγωγή[]
In mathematics, any vector space, V, has a corresponding dual vector space (or just dual space for short) consisting of all linear functionals on V.
Dual vector spaces for finite-dimensional vector spaces can be used for studying tensors.
When applied to vector spaces of functions (which are typically infinite-dimensional), dual spaces are employed for defining and studying concepts like measures, distributions, and Hilbert spaces. Consequently, the dual space is an important concept in the study of functional analysis.
There are two types of dual spaces:
- the algebraic dual space, and the
- continuous dual space.
The algebraic dual space is defined for all vector spaces. When defined for a topological vector space there is a subspace of this dual space, corresponding to continuous linear functionals, which constitutes a continuous dual space.
Algebraic dual space[]
Given any vector space V over a field F, the dual space V* is defined as the set of all linear maps (linear functionals)
- .
The dual space V* itself becomes a vector space over F when equipped with the following addition and scalar multiplication:
for all φ, ψ ∈ V*, x ∈ V, and a ∈ F.
Elements of the algebraic dual space V* are sometimes called covectors or one-forms.
The pairing of a functional φ in the dual space V* and an element x of V is sometimes denoted by a bracket: φ(x) = [φ,x] or φ(x) = ⟨φ,x⟩.
The pairing defines a nondegenerate bilinear mapping[1] [·,·] : V* × V → F.
Finite-dimensional case[]
If V is finite-dimensional, then V* has the same dimension as V. Given a basis e1, ..., en in V, it is possible to construct a specific basis in V*, called the dual basis.
This dual basis is a set e1, ..., en of linear functionals on V, defined by the relation
for any choice of coefficients ci ∈ F. In particular, letting in turn each one of those coefficients be equal to one and the other coefficients zero, gives the system of equations
- where: δij is the Kronecker delta symbol.
For example if V is R2, and its basis chosen to be e1 = (1, 0), e2 = (0, 1), then e1 and e2 are one-forms (functions which map a vector to a scalar) such that e1(e1) = 1, e1(e2) = 0, e2(e1) = 0, and e2(e2) = 1}}. (Note: The superscript here is the index, not an exponent).
In particular, if we interpret Rn as the space of columns of n real numbers, its dual space is typically written as the space of rows of n real numbers. Such a row acts on Rn as a linear functional by ordinary matrix multiplication.
If V consists of the space of geometrical vectors (arrows) in the plane, then the level curves of an element of V* form a family of parallel lines in V. So an element of V* can be intuitively thought of as a particular family of parallel lines covering the plane. To compute the value of a functional on a given vector, one needs only to determine which of the lines the vector lies on. Or, informally, one "counts" how many lines the vector crosses.
More generally, if V is a vector space of any dimension, then the level sets of a linear functional in V* are parallel hyperplanes in V, and the action of a linear functional on a vector can be visualized in terms of these hyperplanes.
Infinite-dimensional case[]
If V is not finite-dimensional but has a basis[2] eα indexed by an infinite set A, then the same construction as in the finite-dimensional case yields linearly independent elements eα (α ∈ A) of the dual space, but they will not form a basis.
Consider, for instance, the space R∞, whose elements are those sequences of real numbers which have only finitely many non-zero entries, which has a basis indexed by the natural numbers N: for i ∈ N, ei is the sequence which is zero apart from the ith term, which is one. The dual space of R∞ is RN, the space of all sequences of real numbers: such a sequence (an) is applied to an element (xn) of R∞ to give the number ∑anxn, which is a finite sum because there are only finitely many nonzero xn. The dimension of R∞ is countably infinite, whereas RN does not have a countable basis.
This observation generalizes to any[2] infinite-dimensional vector space V over any field F: a choice of basis {eα : α ∈ Aidentifies V} with the space (FA)0 of functions f : A → F such that fα = f(α) is nonzero for only finitely many α ∈ A, where such a function f is identified with the vector
in V (the sum is finite by the assumption on f, and any v ∈ V may be written in this way by the definition of the basis).
The dual space of V may then be identified with the space FA of all functions from A to F: a linear functional T on V is uniquely determined by the values θα = T(eα) it takes on the basis of V, and any function θ : A → F (with θ(α) = θα}}) defines a linear functional T on V by
Again the sum is finite because fα is nonzero for only finitely many α.
Note that (FA)0 may be identified (essentially by definition) with the direct sum of infinitely many copies of F (viewed as a 1-dimensional vector space over itself) indexed by A, i.e., there are linear isomorphisms
On the other hand FA is (again by definition), the direct product of infinitely many copies of F indexed by A, and so the identification
is a special case of a general result relating direct sums (of modules) to direct products.
Thus if the basis is infinite, then the algebraic dual space is always of larger dimension than the original vector space. This is in marked contrast to the case of the continuous dual space, discussed below, which may be isomorphic to the original vector space even if the latter is infinite-dimensional.
Bilinear products and dual spaces[]
If V is finite-dimensional, then V is isomorphic to V*. But there is in general no natural isomorphism between these two spaces.
Any bilinear form ⟨•,•⟩ on V gives a mapping of V into its dual space via
where the right hand side is defined as the functional on V taking each w ∈ V to ⟨v,w⟩. In other words, the bilinear form determines a linear mapping
defined by
If the bilinear form is nondegenerate, then this is an isomorphism onto a subspace of V*. If V is finite-dimensional, then this is an isomorphism onto all of V*. Conversely, any isomorphism Φ from V to a subspace of V* (resp., all of V*) defines a unique nondegenerate bilinear form ⟨•,•⟩Φ on V by
Thus there is a one-to-one correspondence between isomorphisms of V to subspaces of (resp., all of) V* and nondegenerate bilinear forms on V.
If the vector space V is over the complex field, then sometimes it is more natural to consider sesquilinear forms instead of bilinear forms. In that case, a given sesquilinear form ⟨•,•⟩ determines an isomorphism of V with the complex conjugate of the dual space
The conjugate space V* can be identified with the set of all additive complex-valued functionals f : V → C such that
Injection into the double-dual[]
There is a natural homomorphism Ψ from V into the double dual V**, defined by (Ψ(v))(φ) = φ(v) for all v ∈ V, φ ∈ V*. This map Ψ is always injective;[2] it is an isomorphism if and only if V is finite-dimensional. Indeed, the isomorphism of a finite-dimensional vector space with its double dual is an archetypal example of a natural isomorphism. Note that infinite-dimensional Hilbert spaces are not a counterexample to this, as they are isomorphic to their continuous duals, not to their algebraic duals.
Transpose of a linear map[]
If f : V → W is a linear map, then the transpose (or dual) f* : W* → V* is defined by
for every φ ∈ W*. The resulting functional f*(φ) in V* is called the pullback of φ along f.
The following identity holds for all φ ∈ W* and v ∈ V:
where the bracket [•,•] on the left is the duality pairing of V with its dual space, and that on the right is the duality pairing of W with its dual. This identity characterizes the transpose,[3] and is formally similar to the definition of the adjoint.
The assignment f ↦ f* produces an injective linear map between the space of linear operators from V to W and the space of linear operators from W* to V*; this homomorphism is an isomorphism if and only if W is finite-dimensional. If V = W then the space of linear maps is actually an algebra under composition of maps, and the assignment is then an antihomomorphism of algebras, meaning that (fg)* = g*f*. In the language of category theory, taking the dual of vector spaces and the transpose of linear maps is therefore a contravariant functor from the category of vector spaces over F to itself. Note that one can identify (f*)* with f using the natural injection into the double dual.
If the linear map f is represented by the matrix A with respect to two bases of V and W, then f* is represented by the transpose matrix AT with respect to the dual bases of W* and V*, hence the name. Alternatively, as f is represented by A acting on the left on column vectors, f* is represented by the same matrix acting on the right on row vectors. These points of view are related by the canonical inner product on Rn, which identifies the space of column vectors with the dual space of row vectors.
Quotient spaces and annihilators[]
Let S be a subset of V. The annihilator of S in V*, denoted here So, is the collection of linear functionals f ∈ V* such that [f, s] = 0 for all s ∈ S. That is, So consists of all linear functionals f : V → F such that the restriction to S vanishes: f|S = 0.
The annihilator of a subset is itself a vector space. In particular, ∅o = V* is all of V* (vacuously), whereas Vo = 0 is the zero subspace. Furthermore, the assignment of an annihilator to a subset of V reverses inclusions, so that if S ⊂ T ⊂ V, then
Moreover, if A and B are two subsets of V, then
and equality holds provided V is finite-dimensional. If Ai is any family of subsets of V indexed by i belonging to some index set I, then
In particular if A and B are subspaces of V, it follows that
If V is finite-dimensional, and W is a vector subspace, then
after identifying W with its image in the second dual space under the double duality isomorphism V ≈ V**. Thus, in particular, forming the annihilator is a Galois connection on the lattice of subsets of a finite-dimensional vector space.
If W is a subspace of V then the quotient space V/W is a vector space in its own right, and so has a dual. By the first isomorphism theorem, a functional f : V → F factors through V/W if and only if W is in the kernel of f. There is thus an isomorphism
As a particular consequence, if V is a direct sum of two subspaces A and B, then V* is a direct sum of Ao and Bo.
Continuous dual space ≡ Dual space of a topological vector space[]
When dealing with topological vector spaces, one is typically only interested in the continuous linear functionals from the space into the base field (or ). This gives rise to the notion of the "continuous dual space" or "topological dual" which is a linear subspace of the algebraic dual space , denoted by . For any finite-dimensional normed vector space or topological vector space, such as Euclidean n-space, the continuous dual and the algebraic dual coincide. This is however false for any infinite-dimensional normed space, as shown by the example of discontinuous linear maps. Nevertheless in the theory of topological vector spaces the terms "continuous dual space" and "topological dual space" are rarely used, as a rule they are replaced by "dual space", since there is no serious need to consider discontinuous maps in this field.
For a topological vector space its continuous dual space,[4] or topological dual space,[5] or just dual space[4][5][6][7] (in the sense of the theory of topological vector spaces) is defined as the space of all continuous linear functionals .
There is a standard construction for introducing topology on the continuous dual of a topological vector space : each given class of bounded subsets in defines a topology on of uniform convergence on sets from , or what is the same a topology generated by seminorms of the form
where is a continuous linear functional on , and runs over the class .
This means that a net of functionals tends to a functional in if and only if
Usually (but not necessarily) the class is supposed to satisfy the following conditions:
- each point of belongs to some set
- each two sets and are contained in some set :
- is closed under the operation of multiplication by scalars:
If these requirements are fulfilled then the coresponding topology on is Hausdorff and the sets
form its local base.
Here are the three most important special cases.
- The strong topology on is the topology of uniform convergence on bounded subsets in (so here can be chosen as the class of all bounded subsets in ). If is a normed vector space (e.g., a Banach space or a Hilbert space) then the strong topology on is normed (in fact a Banach space if the field of scalars is complete), with the norm
- The stereotype topology on is the topology of uniform convergence on totally bounded sets in (so here can be chosen as the class of all totally bounded subsets in ).
- The weak topology on is the topology of uniform convergence on finite subsets in (so here can be chosen as the class of all finite subsets in ).
Each of these three choices of topology on leads to a variant of reflexivity property for topological vector spaces.
Examples[]
Let 1 < p < ∞ be a real number and consider the Banach space ℓ p of all sequences a = (an) for which
is finite. Define the number q by 1/p + 1/q = 1. Then the continuous dual of ℓ p is naturally identified with ℓ q: given an element φ ∈ (ℓ p)′, the corresponding element of ℓ q is the sequence (φ(en)) where en denotes the sequence whose n-th term is 1 and all others are zero. Conversely, given an element a = (an) ∈ ℓ q, the corresponding continuous linear functional φ on ℓ p is defined by φ(b) = ∑n anbn for all b = (bn) ∈ ℓ p (see Hölder's inequality).
In a similar manner, the continuous dual of ℓ 1 is naturally identified with ℓ ∞ (the space of bounded sequences). Furthermore, the continuous duals of the Banach spaces c (consisting of all convergent sequences, with the supremum norm) and c0 (the sequences converging to zero) are both naturally identified with ℓ 1.
By the Riesz representation theorem, the continuous dual of a Hilbert space is again a Hilbert space which is anti-isomorphic to the original space. This gives rise to the bra-ket notation used by physicists in the mathematical formulation of quantum mechanics.
Transpose of a continuous linear map[]
If T : V → W is a continuous linear map between two topological vector spaces, then the (continuous) transpose T′ : W′ → V′ is defined by the same formula as before:
The resulting functional T′(φ) is in V′. The assignment T → T′ produces a linear map between the space of continuous linear maps from V to W and the space of linear maps from W′ to V′. When T and U are composable continuous linear maps, then
When V and W are normed spaces, the norm of the transpose in L(W′, V′) is equal to that of T in L(V, W). Several properties of transposition depend upon the Hahn–Banach theorem. For example, the bounded linear map T has dense range if and only if the transpose T′ is injective.
When T is a compact linear map between two Banach spaces V and W, then the transpose T′ is compact. This can be proved using the Arzelà–Ascoli theorem.
When V is a Hilbert space, there is an antilinear isomorphism iV from V onto its continuous dual V′. For every bounded linear map T on V, the transpose and the adjoint operators are linked by
When T is a continuous linear map between two topological vector spaces V and W, then the transpose T′ is continuous when W′ and V′ are equipped with"compatible" topologies: for example when, for X = V and X = W, both duals X′ have the strong topology β(X′, X) of uniform convergence on bounded sets of X, or both have the weak-∗ topology σ(X′, X) of pointwise convergence on X. The transpose T′ is continuous from β(W′, W) to β(V′, V), or from σ(W′, W) to σ(V′, V).
Annihilators[]
Assume that W is a closed linear subspace of a normed space V, and consider the annihilator of W in V′,
Then, the dual of the quotient V / W can be identified with W⊥, and the dual of W can be identified with the quotient V′ / W⊥.
Indeed, let P denote the canonical surjection from V onto the quotient V / W ; then, the transpose P′ is an isometric isomorphism from (V / W )′ into V′, with range equal to W⊥. If j denotes the injection map from W into V, then the kernel of the transpose j′ is the annihilator of W:
and it follows from the Hahn-Banach theorem that j′ induces an isometric isomorphism V′ / W⊥ → W′.
Further properties[]
If the dual of a normed space V is separable, then so is the space V itself. The converse is not true: for example the space ℓ 1 is separable, but its dual is ℓ ∞ is not.
Double dual[]
In analogy with the case of the algebraic double dual, there is always a naturally defined continuous linear operator Ψ : V → V′′ from a normed space V into its continuous double dual V′′, defined by
As a consequence of the Hahn–Banach theorem, this map is in fact an isometry, meaning ||Ψ(x)|| = ||x|| for all x in V. Normed spaces for which the map Ψ is a bijection are called reflexive.
When V is a topological vector space, one can still define Ψ(x) by the same formula, for every x ∈ V, however several difficulties arise. First, when V is not locally convex, the continuous dual may be equal to {0} and the map Ψ trivial. However, if V is Hausdorff and locally convex, the map Ψ is injective from V to the algebraic dual V′* of the continuous dual, again as a consequence of the Hahn–Banach theorem.[8]
Second, even in the locally convex setting, several natural vector space topologies can be defined on the continuous dual V′, so that the continuous double dual V′′ is not uniquely defined as a set. Saying that Ψ maps from V to V′′, or in other words, that Ψ(x) is continuous on V′ for every x ∈ V, is a reasonable minimal requirement on the topology of V′, namely that the evaluation mappings
be continuous for the chosen topology on V′. Further, there is still a choice of a topology on V′′, and continuity of Ψ depends upon this choice. As a consequence, defining reflexivity in this framework is more involved than in the normed case.
The mind blowing intuition behind dual spaces[]
The dual is intuitively the space of “rulers” (or measurement-instruments) of our vector space. Its elements measure vectors. This is what makes the dual space and its relatives so important in Differential Geometry, for instance. This immediately motivates the study of the dual space.
This also happens to explain intuitively some facts. For instance, the fact that there is no canonical isomorphism between a vector space and its dual can then be seen as a consequence of the fact that rulers need scaling, and there is no canonical way to provide one scaling for space.
However, if we were to measure the measure-instruments, how could we proceed? Is there a canonical way to do so? Well, if we want to measure our measures, why not measure them by how they act on what they are supposed to measure? We need no bases for that. This justifies intuitively why there is a natural embedding of the space on its bidual. (Note, however, that this fails to justify why it is an isomorphism in the finite-dimensional case).
This is the intuition behind the relation: u(φ) = φ(u). Here u is the “ruler” by which we “measure” elements of the dual space V*. And we “calilbrate” that ruler by taking into account how φ (the “ruler” of V) “measures” elements u of V.
Υποσημειώσεις[]
- ↑ In many areas, such as quantum mechanics, ⟨·,·⟩ is reserved for a sesquilinear form defined on Δεν μπόρεσε να γίνει ανάλυση του όρου. (Λάθος σύνταξης): {\displaystyle V × V} .
- ↑ 2,0 2,1 2,2 Several assertions in this article require the axiom of choice for their justification. The axiom of choice is needed to show that an arbitrary vector space has a basis: in particular it is needed to show that RN has a basis. It is also needed to show that the dual of an infinite-dimensional vector space V is nonzero, and hence that the natural map from V to its double dual is injective.
- ↑ Πρότυπο:Harvtxt
- ↑ 4,0 4,1 Πρότυπο:Harvtxt
- ↑ 5,0 5,1 Πρότυπο:Harvtxt
- ↑ Πρότυπο:Harvtxt
- ↑ Πρότυπο:Harvtxt
- ↑ If V is locally convex but not Hausdorff, the kernel of Ψ is the smallest closed subspace containing {0}.
Εσωτερική Αρθρογραφία[]
- Duality
- projective Duality
- Pontryagin duality
- Reciprocal lattice – dual space basis, in crystallography
- Covariance and contravariance of vectors
- Dual norm
- Ευκλείδειος Χώρος
- Τανυστικός Χώρος
- Εφαπτομενικός Χώρος
- Δυϊκότητα
Βιβλιογραφία[]
Ιστογραφία[]
- Ομώνυμο άρθρο στην Βικιπαίδεια
- Ομώνυμο άρθρο στην Livepedia
- slideplayer.com/slide/
- Duality of Vector Spaces, Solmaz
|
Αν και θα βρείτε εξακριβωμένες πληροφορίες "Οι πληροφορίες αυτές μπορεί πρόσφατα Πρέπει να λάβετε υπ' όψη ότι Επίσης, |
- Μην κάνετε χρήση του περιεχομένου της παρούσας εγκυκλοπαίδειας
αν διαφωνείτε με όσα αναγράφονται σε αυτήν
- Όχι, στις διαφημίσεις που περιέχουν απαράδεκτο περιεχόμενο (άσεμνες εικόνες, ροζ αγγελίες κλπ.)










![{\displaystyle [\Phi _{\langle \cdot ,\cdot \rangle }(v),w]=\langle v,w\rangle .}](https://services.fandom.com/mathoid-facade/v1/media/math/render/svg/e733a7bc127b039f0292c0f7db07f1e41c366e41)
![{\displaystyle \langle v,w\rangle _{\Phi }=(\Phi (v))(w)=[\Phi (v),w].\,}](https://services.fandom.com/mathoid-facade/v1/media/math/render/svg/a76271f50176328a0a4169a57ccf6609e764c9c4)



![{\displaystyle [f^{*}(\varphi ),\,v]=[\varphi ,\,f(v)],}](https://services.fandom.com/mathoid-facade/v1/media/math/render/svg/bb9cf5f61339d45e7e9e73b02d6565abe3512dbd)