Χώρος Minkowski

Μιγγόσειος Χώρος

Minkowski Space

Χώρος Minkowski Σύστημα Συντεταγμένων

Χώρος Minkowski Σύστημα Συντεταγμένων

Πυθαγόρειο Θεώρημα
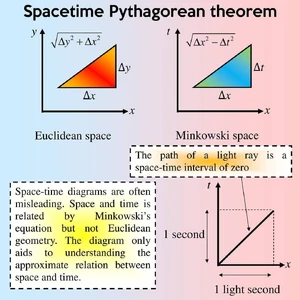
Ειδική Σχετικότητα
Κώνος Φωτός

Μαθηματικά Γραμμική Άλγεβρα

---

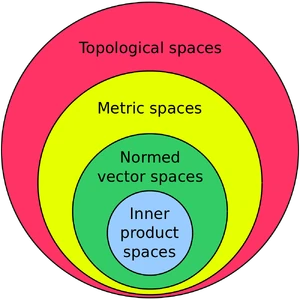
Μαθηματικός Χώρος Φυσικός Χώρος Τοπολογικός Χώρος Διανυσματικός Χώρος Μετρικός Χώρος Χώρος Hilbert

---

Ευκλείδειος Χώρος Χώρος Riemann Χώρος Lobachevsky

Κώνος Φωτός σε δισδιάστατη μορφή

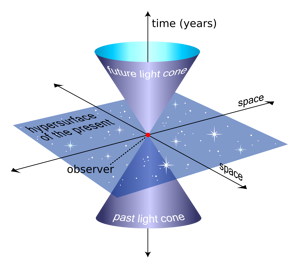
Κώνος Φωτός σε τρισδιάστατη μορφή

Σχετικιστική Φυσική Σχετικιστική Πεδιακή Θεωρία Σχετικιστική Κλασσική Μηχανική Σχετικιστική Κβαντική Μηχανική Ειδική Σχετικότητα Γενική Σχετικότητα Χωροχρόνος Χώρος Minkowski Σχετικιστικοί Φυσικοί Γης

Einstein Albert Ειδική Σχετικότητα Γενική Σχετικότητα

Μετατόπιση Χώρος Minkowski

Κώνος Φωτός
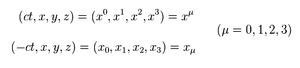
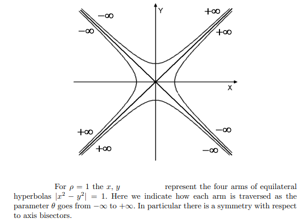
Υπερβολή

- Ένας Χωρόχρονος τεσσάρων διαστάσεων.

Ετυμολογία

Το όνομα "Μιγγόσκειος" σχετίζεται ετυμολογικά με το όνομα "Minkowski".

"Μιγγόσειος" είναι ο εξελληνισμός του όρου "χώρος Minkowski" σε αντιστοιχία με

• το "Ευκλείδειος" που αντιστοιχεί στο "χώρος Euclid" (γλωσσικό αντιδάνειο)
• το "Νευτώνεια" που αντιστοιχεί στo "Μηχανική Newton"
• το "Αβελιανή" που αντιστοιχεί στo "ομάδα Abel"
• το "Χαμιλτονιανή" που αντιστοιχεί στo "συνάρτηση Hamilton"
• το "Λαγρασιανή" που αντιστοιχεί στo "συνάρτηση Lagrange"
• το "Λαπλασιανή" που αντιστοιχεί στo "τελεστής Laplace"
• το "κυρκόνιο" που αντιστοιχεί στο σωματίδιο "quark"

Καλό είναι να εξελληνίζουμε τους πολύ συνήθεις όρους της Αγγλικής Βιβλιογραφίας όταν γράφουμε Ελληνικά για να διευκολύνεται ο αναγνώστης στην απομνημόνευση διάφορων επιστημονικών νεολογισμών.

Εισαγωγή

Χρησιμοποιείται στην Ειδική Σχετικότητα.

Ο Minkowski χρησιμοποίησε ένα γεωμετρικό χώρο, κάθε σημείο του οποίου αντιπροσωπεύει ένα γεγονός.

Μία καλύτερη κατανόηση του χώρου αυτού γίνεται με την χρήση του κώνου φωτός.

Οι δύο ευθείες που διέρχονται από την αρχή των αξόνων είναι οι διχοτόμοι των αντίστοιχων γωνιών και παίζουν σημαντικό ρόλο.

Ένα γεγονός, είναι δυνατό να συμβεί όχι μόνο στην αρχή των αξόνων, αλλά και σε κάποιο άλλο σημείο του Χώρου και του Χρόνου.

Αν υποθέσουμε ότι στην αρχή των αξόνων συμβαίνει ένα γεγονός, τότε

• Οποιοδήποτε γεγονός συμβαίνει άνωθεν του άξονα x ανήκει στο μέλλον.

• Οποιοδήποτε γεγονός συμβαίνει οτιδήποτε κάτωθεν του άξονα x, θα ανήκει στο παρελθόν.

Χρησιμοποιείται η εξής ορολογία:

• Οποιοδήποτε γεγονός συμβαίνει μέσα στην πράσινη περιοχή, αποκαλείται χρονοειδές.

• Οποιοδήποτε γεγονός συμβαίνει στην κυανή περιοχή λέγεται χωροειδές.

• Οποιοδήποτε γεγονός συμβαίνει στις δύο κίτρινες ευθείες, λέγεται φωτοειδές.

Σχετικότητα

Όμως με την επιλογή ενός άλλου συστήματος αναφοράς (Αδρανειακό Σύστημα), το ότι θεωρήθηκε ως παρόν, παρελθόν και μέλλον, γίνεται σχετικό.

• Παρατηρώντας από το Αδρανειακό Σύστημα, με άξονες (x, ct), το γεγονός Γ ανήκει στο μέλλον, ενώ
• Παρατηρώντας από το Αδρανειακό Σύστημα, με άξονες (x', ct') το γεγονός Γ ανήκει στο παρελθόν.

Δομή

Formally, Minkowski space is a four-dimensional real vector space equipped with a nondegenerate, symmetric bilinear form with signature (−,+,+,+) (Some may also prefer the alternative signature (+,−,−,−); in general, mathematicians and general relativists prefer the former while particle physicists tend to use the latter.) In other words, Minkowski space is a pseudo-Euclidean space with n = 4 and n − k = 1 (in a broader definition any n > 1 is allowed).

Elements of Minkowski space are called events or four-vectors. Minkowski space is often denoted R^1,3 to emphasize the signature, although it is also denoted M⁴ or simply M. It is perhaps the simplest example of a pseudo-Riemannian manifold.

Compared to the more general concept of spacetime in general relativity, where a Lorentzian manifold M is equipped with a metric tensor g, which is a symmetric bilinear form on each tangent space, the Minkowski space is the relatively simple special case where every tangent space is the space itself, and its metric tensor, called the Minkowski tensor η, is a single symmetric bilinear form. See also below.

Minkowski tensor

The Minkowski tensor is the metric tensor of Minkowski space.

It is a symmetric bilinear form (type-(0,2) tensor) similar to the Euclidean inner product, but it is used to describe a different geometry; the geometry is usually associated with relativity. Let M be a 4-dimensional real vector space.

The Minkowski tensor is a map 1=η : M × M → R

(i.e. given any two vectors v, w in M, η(v,w) is a real number, called the magnitude of the vector) which satisfies properties (1), (2), and (3) listed here, as well as property (4) given below:

1.	bilinear	η(au+v, w) = aη(u,w) + η(v,w) for all a ∈ R and u, v, w in M.
2.	symmetric	η(v,w) = η(w,v) for all v, w ∈ M.
3.	nondegenerate	if η(v,w) = 0 for all w ∈ M then v = 0.

Just as in Euclidean space, two vectors v and w are said to be orthogonal if η(v,w) = 0. Minkowski space differs by including hyperbolic-orthogonal events in case v and w span a plane where η takes negative values. This difference is clarified by comparing the Euclidean structure of the ordinary complex number plane to the structure of the plane of split-complex numbers.

The Minkowski norm of a vector v is defined by

$\|v\| = \sqrt{|\eta(v,v)|}.$

This is not a norm (and not even a seminorm) in the usual sense because it fails to be subadditive, but it does define a useful generalization of the notion of length to Minkowski space. In particular, a vector v is called a unit vector if ||v|| = 1 (i.e., η(v,v) = ±1). A basis for M consisting of mutually orthogonal unit vectors is called an orthonormal basis.

For a given inertial frame, an orthonormal basis in space, combined by the unit time vector, forms an orthonormal basis in Minkowski space. Furthermore, the number of positive and negative unit vectors in any such basis is a fixed pair of numbers, equal to the signature of the tensor. This is Sylvester's law of inertia.

Then the fourth condition on η can be stated:

4.

signature

The bilinear form η has signature (−,+,+,+) or (+,−,−,−).

Which signature is used is a matter of convention. Both are fairly common.

The magnitude of the difference between two vectors is called the spacetime interval.

Standard basis

A standard basis for Minkowski space is a set of four mutually orthogonal vectors { e₀, e₁, e₂, e₃ } such that

$-\eta(e_0, e_0) = \eta(e_1, e_1) = \eta(e_2, e_2) = \eta(e_3, e_3) = 1 .$

These conditions can be written compactly in the following form:

$\eta(e_\mu, e_\nu) = \eta_{\mu \nu}$

where μ and ν run over the values 0, 1, 2, 3 and the matrix [η_μν] is given by

$[\eta_{\mu\nu}] = \begin{pmatrix}-1&0&0&0\\0&1&0&0\\0&0&1&0\\0&0&0&1\end{pmatrix}$ .

(As was previously noted, sometimes the opposite sign convention is preferred.)

Relative to a standard basis, the components of a vector v are written (v⁰, v¹, v², v³) and we use the Einstein notation to write 1=v = v^μe_μ.

The component v⁰ is called the timelike component of v while the other three components are called the spatial components.

In terms of components, the inner product between two vectors v and w is given by

$\eta(v, w) = \eta_{\mu \nu} v^\mu w^\nu = - v^0 w^0 + v^1 w^1 + v^2 w^2 + v^3 w^3$

and

$\eta(v, v) = \eta_{\mu \nu} v^\mu v^\nu = - (v^0)^2 + (v^1)^2 + (v^2)^2 + (v^3)^2$ (magnitude of v)

Εσωτερική Αρθρογραφία

• Ευκλείδειος Χώρος
• Ρημάννιος Χώρος

Ιστογραφία

• Ομώνυμο άρθρο στην Βικιπαίδεια
• Ομώνυμο άρθρο στην Livepedia
• Χώρος_Minkowski
• ΛΥΚΕΡΙ∆ΗΣ
• [ ]

Κίνδυνοι Χρήσης

Αν και θα βρείτε εξακριβωμένες πληροφορίες σε αυτήν την εγκυκλοπαίδεια ωστόσο, παρακαλούμε να λάβετε σοβαρά υπ' όψη ότι η "Sciencepedia" δεν μπορεί να εγγυηθεί, από καμιά άποψη, την εγκυρότητα των πληροφοριών που περιλαμβάνει. "Οι πληροφορίες αυτές μπορεί πρόσφατα να έχουν αλλοιωθεί, βανδαλισθεί ή μεταβληθεί από κάποιο άτομο, η άποψη του οποίου δεν συνάδει με το "επίπεδο γνώσης" του ιδιαίτερου γνωστικού τομέα που σας ενδιαφέρει." Πρέπει να λάβετε υπ' όψη ότι όλα τα άρθρα μπορεί να είναι ακριβή, γενικώς, και για μακρά χρονική περίοδο, αλλά να υποστούν κάποιο βανδαλισμό ή ακατάλληλη επεξεργασία, ελάχιστο χρονικό διάστημα, πριν τα δείτε. Επίσης, Οι διάφοροι "Εξωτερικοί Σύνδεσμοι (Links)" (όχι μόνον, της Sciencepedia αλλά και κάθε διαδικτυακού ιστότοπου (ή αλλιώς site)), αν και άκρως απαραίτητοι, είναι αδύνατον να ελεγχθούν (λόγω της ρευστής φύσης του Web), και επομένως είναι ενδεχόμενο να οδηγήσουν σε παραπλανητικό, κακόβουλο ή άσεμνο περιεχόμενο. Ο αναγνώστης πρέπει να είναι εξαιρετικά προσεκτικός όταν τους χρησιμοποιεί.

- Μην κάνετε χρήση του περιεχομένου της παρούσας εγκυκλοπαίδειας
αν διαφωνείτε με όσα αναγράφονται σε αυτήν

---

>>Διαμαρτυρία προς την wikia<<

- Όχι, στις διαφημίσεις που περιέχουν απαράδεκτο περιεχόμενο (άσεμνες εικόνες, ροζ αγγελίες κλπ.)

---